TOP 7 Famous Arab & Persian Scientists and their Inventions during Islamic Golden Age

Muslim scientists and inventors, including Arabs, Persians and Turks, were probably hundreds of years ahead of their counterparts in the European Middle Ages. They drew influence from Aristotelian philosophy and Neo-platonists, as well as Euclid, Archimedes, Ptolemy and others. The muslims made innumerable discoveries and wrote countless books about medicine, surgery, physics, chemistry, philosophy, astrology, geometry and various other fields.

Today’s article discusses the most famous muslim scientists and inventors and their wonderful inventions.

1) Abu Nasr Al-Farabi

Abu Nasr Muhammad al-Farabi was one the earliest Islamic intellectuals who was instrumental in transmitting the doctrines of Plato and Aristotle to the Muslim world. He had a considerable influence on the later Islamic philosophers such as Avicenna. He was an outstanding linguist who translated the Greek works of Aristotle and Plato and made considerable additions to them of his own. He earned the nickname Mallim-e-Sani, which is translated as “second master” or “second teacher”.

Early Life:

Al-Farabi completed his earlier education at Farab and Bukhara. Later on he went to Baghdad for higher studies where he studied and worked for a long time. During this period he acquired mastery of several languages as well as various branches of knowledge and technology. Farabi contributed considerably to science, philosophy, logic, sociology, medicine, mathematics and music. His major contributions were in philosophy, logic and sociology and for which he stands out as an Encyclopedist.

Contributions and Achievements:

As a philosopher, Farabi was the first to separate philosophy from theology. It is difficult to find a philosopher both in Muslim and Christian world from Middle Ages onwards who has not been influenced by his views. He believed in a Supreme Being who had created the world through the exercise of balanced intelligence. He also asserted this same rational faculty to be the sole part of the human being that is immortal, and thus he set as the paramount human goal the development of that rational faculty. He gave considerably more attention to political theory compared to any Islamic philosopher.

Later in his work, Farabi laid down in Platonic fashion the qualities necessary for the ruler. He stated that a ruler should be inclined to rule by a good quality of a native character and exhibit the right attitude for such rule. At the heart of Al-Farabi’s political philosophy is the concept of happiness, in which people cooperate to gain contentment. He followed the Greek example and the highest rank of happiness was allocated to his ideal sovereign whose soul was ‘united as it were with the Active Intellect’. Farabi served as a tremendous source of aspiration for intellectuals of the middle ages and made enormous contributions to the knowledge of his day, paving the way for the later philosopher and thinkers of the Muslim world.

Farabian epistemology has both a Neoplatonic and an Aristotelian dimension. The best source for Farabi’s classification of knowledge is his Kitab ihsa al-ulum. This work neatly illustrates Farabi’s beliefs, both esoteric and exoteric. Through all of them runs a primary Aristotelian stress on the importance of knowledge. Thus al-Farabi’s epistemology, from what has been described may be said to be encyclopedic in range and complex in articulation, using both a Neoplatonic and an Aristotelian voice.

Farabi also participated in writing books on early Muslim sociology and a notable book on music titled Kitab al-Musiqa (The Book of Music). This book is, in reality, a study of the theory of Persian music of his day, although in the West it has been introduced as a book on Arab music. He invented several musical instruments, besides contributing to the knowledge of musical notes. It has been reported that he could play his instrument so well as to make people laugh or weep at will. Farabi’s treatise Meanings of the Intellect dealt with music therapy, where he discussed the therapeutic effects of music on the soul.

Later Life:

Farabi traveled to many distant lands throughout his life and gained many experiences. As a result, he made many contributions for which he is still remembered and acknowledged. Despite facing many hardships he worked with full dedication and made his name among the popular scientists of history. He died a bachelor in Damascus in 339 A.H. /950 A.D. at the age of 80 years.

2) Al-Battani

Al-Battani is sometimes known by a Latinized version of his name, being Albategnius, Albategni or Albatenius. His full name was Abu Abdallah Mohammad ibn Jabir ibn Sinan al-Raqqi al-Harrani al-Sabi al-Battani.

Al-Battani’s father was Jabir ibn Sinan al-Harrani who had a high reputation as an instrument maker in Harran. The name makes the identification certain that al-Battani himself was skilled in making astronomical instruments and there is a good indication that he learnt these skills from his father.

Early Life and Career:

Abdallah Muhammad Ibn Jabir Ibn Sinan al-Battani al-Harrani was born around 858 C.E. in Harran. Battani was first educated by his father Jabir Ibn San’an al-Battani, who also was a well-known scientist. He then moved to Raqqa, situated on the bank of the Euphrates, where he received an advanced education and later on flourished as a scholar. At the beginning of the 9th century, he migrated to Samarra, where he worked till the end of his life. His family had been members of the Sabian sect, a religious sect of star worshippers from Harran. Being worshipers of the stars meant that the Sabians had a strong motivation for the study of astronomy. Al-Battani, unlike Thabit, another mathematician from his home town, was not a believer in the Sabian religion. His name “Abu Abdallah Mohammad” indicates that he was certainly a Muslim.

Al-Battani made remarkably accurate astronomical observations at Antioch and ar-Raqqah in Syria. The town of ar-Raqqah, where most of al-Battani’s observations were made, became prosperous when Caliph Harun al-Rashid built several palaces there.

The Fihrist, who wrote a tenth-century survey of Muslim culture, describes al-Battani as one of the most famous observers and a leader in geometry, theoretical and practical astronomy, and astrology. He composed work on astronomy, with tables, containing his own observations of the sun and moon and a more accurate description of their motions than that given in Ptolemy’s “Almagest”.

The main achievements of al-Battani’s are:

• He cataloged 489 stars.

• He refined the existing values for the length of the year, which he gave as 365 days 5 hours 46 minutes 24 seconds, and of the seasons.

• He calculated 54.5″ per year for the precession of the equinoxes and obtained the value of 23° 35′ for the inclination of the ecliptic.

Rather than using geometrical methods, as other scientists had done, al-Battani used trigonometric methods which were an important advancement. Al-Battani showed that the farthest distance of the Sun from the Earth varies and, as a result, annular eclipses of the Sun are possible as well as total eclipses. Al-Battani is important in the development of science for a number of reasons, but one of these must be the large influence his work had on scientists such as Tycho Brahe, Kepler, Galileo and Copernicus.

Death:

Historians all agree that Al-Battani passed away in 317 H. /929 A.D., near the city of Moussul in Iraq. He was regarded as one of the most famous Arab astronomers. He dedicated all his life until his death to the observation of planets and stars.

3)Avicenna

Also popularly known as ‘Avicenna’, Ibn Sina was indeed a true polymath with his contributions ranging from medicine, psychology and pharmacology to geology, physics, astronomy, chemistry and philosophy. He was also a poet, an Islamic scholar and theologian.His most important contribution to medical science was his famous book Al Qanun Fi Al-Tibb (The Canon of Medicine). This book is an immense five volume encyclopedia of medicine containing over a million words. It comprises of medical knowledge available from ancient and Muslim sources. His other major work is “The Book of Healing”, a scientific and philosophical encyclopedia.

Early Life

This great scientist was born in around 980 A.D in the village of Afshana, near Bukhara, now in Uzbekistan, which is also his mother’s hometown. His father, Abdullah an advocate of the Ismaili sect, was from Balkh which is now a part of Afghanistan. Ibn Sina received his early education in his home town and by the age of ten he became a Quran Hafiz (he had memorized the Quran). He had exceptional intellectual skills which enabled him to overtake his teachers at the age of fourteen. During the next few years he devoted himself to Muslim jurisprudence, philosophy and natural science and studied logic, Euclid, and Ptolemy’s Almagest.

Ibn Sina was an extremely religious man. When he was still young, Ibn Sina was highly baffled by the work of Aristotle on metaphysics so much so that he would pray to God to guide him. Finally after reading a manual by a famous philosopher al-Farabi, he found the solutions to his difficulties.

Contributions and Achievements

At the age of sixteen he dedicated all his efforts to learn medicine and by the time he was eighteen gained the status of a reputed physician. During this time he cured Nuh II, Ruler of the Samanids, of an illness in which all the renowned physicians had given up hope. On this great effort, the Amir wished to reward him, and the young physician requested consent to use his exclusively stocked royal library.

On his father’s death, when Ibn Sina was twenty-two years old, he moved to Jurjan near the Caspian Sea where he lectured on logic and astronomy. Here he also met his famous contemporary Abu Rayhan al-Biruni. Later he travelled to Rey and then to Hamadan (both in present day Iran), where he wrote and taught his works. Here he also cured Shams al-Dawla, the Emir of Hamadan, from a severe illness.

From Hamadan, he moved to Isfahan (now in central Iran), where he finished his epic writings. Nevertheless, he continued to travel and too much mental exertion as well as political chaos affected his health. The last decade or so of his life, he spent in the service of a military commander Ala al-Dawla Muhammad. He served him as a physician and general literary and scientific consultant, including during his campaigns. He died during June 1037 A.D, aged 58 and was buried in Hamedan, Iran.

Works

Avicenna’s most important contribution to medical science was his famous book Al Qanun Fi Al-Tibb (The Canon of Medicine), known as the “Canon” in the West. This book was an immense five volume encyclopedia of medicine including over a million words. It comprised of medical knowledge available from ancient and Muslim sources. This book was translated into Latin in the twelfth century and was used as the standard medical text in European universities until the mid-seventeenth century.

His other major work was “The Book of Healing”, a scientific and philosophical encyclopedia. This book was intended to ‘heal’ the soul. It was split into four parts: logic, natural sciences, mathematics and metaphysics. In his book, he developed his own system of logic, Avicennian logic. In astronomy, he proposed that Venus was closer to the Sun than the Earth. He invented an instrument for observing the coordinates of a star. He made several astronomical observations and stated that the stars were self-luminous. In mathematics, Avicenna explained the arithmetical concept and application of the “casting out of nines”. Ibn Sina also contributed to poetry, religion and music. In total, Avicenna wrote over 400 works, of which around 240 have survived.

4) Ibn Battuta

Abu Abdullah Muhammad Ibn Battuta, was a Moroccan Muslim scholar and traveler. He is known for his traveling and going on excursions called the Rihla. His journeys lasted for a period of almost thirty years. This covered nearly the whole of the known Islamic world and beyond, extending from North Africa, West Africa, Southern Europe and Eastern Europe in the West, to the Middle East, Indian subcontinent, Central Asia, Southeast Asia and China in the East, a distance readily surpassing that of his predecessors. After his travel he returned to Morocco and gave his account of the experience to Ibn Juzay.

Early life and Career:

Abu Abdullah Muhammad Ibn Battuta, was born in Tangier, Morocco, on the 24th of February 1304 C.E. (703 Hijra) during the time of the Marinid dynasty. He was commonly known as Shams ad-Din. His family was of Berber origin and had a tradition of service as judges. After receiving an education in Islamic law, he chose to travel. He left is house in June 1325, when he was twenty one years of age and set off from his hometown on a hajj (pilgrimage) to Mecca, a journey that took him 16 months. He did not come back to Morocco for at least 24 years after that. His journey was mostly by land. To reduce the risk of being attacked, he usually chose to join a caravan. In the town of Sfax, he got married. He survived wars, shipwrecks, and rebellions.

He first began his voyage by exploring the lands of the Middle East. Thereafter he sailed down the Red Sea to Mecca. He crossed the huge Arabian Desert and traveled to Iraq and Iran. In 1330, he set of again, down the Red Sea to Aden and then to Tanzania. Then in 1332, Ibn Battuta decided to go to India. He was greeted open heartedly by the Sultan of Delhi. There he was given the job of a judge. He stayed in India for a period of 8 years and then left for China. Ibn Battuta left for another adventure in 1352. He then went south, crossed the Sahara desert, and visited the African kingdom of Mali.

Finally, he returned home at Tangier in 1355. Those who were lodging Ibn Battuta’s grave Western Orient lists could not believe that Ibn Battuta visited all the places that he described. They argued that in order to provide a comprehensive description of places in the Muslim world in such a short time, Ibn Battuta had to rely on hearsay evidence and make use of accounts by earlier travelers.

Ibn Battuta often experienced culture shock in regions he visited. The local customs of recently converted people did not fit his orthodox Muslim background. Among Turks and Mongols, he was astonished at the way women behaved. They were given freedom of speech. He also felt that the dress customs in the Maldives and some sub-Saharan regions in Africa were too revealing.

Death:

After the completion of the Rihla in 1355, little is known about Ibn Battuta’s life. He was appointed a judge in Morocco and died in 1368. Nevertheless, the Rihla provides an important account of many areas of the world in the 14th century.

5) Ibn Rushd

Early Life:

Abu Walid Mohammad Ibn Rushd born in 1128 C.E. in Cordova has been held as one of the greatest thinkers and scientists of the history. A product of twelfth-century Islamic Spain, he set out to integrate Aristotelian philosophy with Islamic thought. A common theme throughout his writings is that there is no inappropriateness between religion and philosophy when both are properly understood.

His contributions to philosophy took many forms, ranging from his detailed commentaries on Aristotle, his defence of philosophy against the attacks of those who condemned it as different to Islam and his construction of a form of Aristotelianism which cleansed it, as far as was possible at the time, of, Neoplatonic influences.

Contributions and Achievements:

Ibn Rushd’s education followed a traditional path, beginning with studies in Hadith, linguistics, jurisprudence and scholastic theology. Throughout his life he wrote extensively on Philosophy and Religion, attributes of God, origin of the universe, Metaphysics and Psychology but he excelled in philosophy and jurisprudence and was nicknamed “the jurisprudent philosopher.” The role of the philosopher in the state was a topic of continual interest for Ibn Rushd.

His thought is genuinely creative and highly controversial, producing powerful arguments that were to puzzle his philosophical successors in the Jewish and Christian worlds. He seems to argue that there are two forms of truth, a religious form and a philosophical form, and that it does not matter if they point in different directions. He also appears to be doubtful about the possibility of personal immortality or of Gods being able to know that particular events have taken place. There is much in his work also which suggests that religion is inferior to philosophy as a means of attaining knowledge, and that the understanding of religion which ordinary believers can have is very different and impoverished when compared with that available to the philosopher.

In philosophy, his most important work Tuhafut al-Tuhafut was written in response to Al-Ghazali’s work. Ibn Rushd was criticized by many Muslim scholars for this book, which, nevertheless, had a deep influence on European thought, at least until the beginning of modern philosophy and experimental science. His views on fate were that man is neither in full control of his destiny nor is it fully predetermined for him. Al Rushd’s longest commentary was, in fact, an original contribution as it was largely based on his analysis including interpretation of Quranic concepts. Ibn Rushd’s summary the opinions (fatwa) of previous Islamic jurists on a variety of issues has continued to influence Islamic scholars to the present day, notably Javed Ahmad Ghamidi.

At the age of 25, Ibn Rushd conducted astronomical observations in Morocco, during which he discovered a previously unobserved star. He was also of the view that the Moon is opaque and obscure, and has some parts which are thicker than others, with the thicker parts receiving more light from the Sun than the thinner parts of the Moon. He also gave one of the first descriptions on sunspots.

Ibn Rushd also made remarkable contributions in medicine. In medicine his well-known book Kitab al-Kulyat fi al-Tibb was written before 1162 A.D Its Latin translation was known as ‘Colliget’. In it Ibn Rushd has thrown light on various aspects of medicine, including the diagnoses, cure and prevention of diseases and several original observations of him.

He wrote at least 67 original works, which included 28 works on philosophy, 20 on medicine, 8 on law, 5 on theology, and 4 on grammar, in addition to his commentaries on most of Aristotle’s works and his commentary on Plato’s The Republic. A careful examination of his works reveals that Ibn Rushd (Averroes) was a deeply Islamic man. As an example, we find in his writing, “Anyone who studies anatomy will increase his faith in the omnipotence and oneness of God the Almighty”. He believed that true happiness for man can surely be achieved through mental and psychological health, and people cannot enjoy psychological health unless they follow ways that lead to happiness in the hereafter, and unless they believe in God and His oneness.

Death:

Ibn Rushd died in Marakesh in 1198 where he was buried. Three months later, his body was moved to Qurtuba, the tribune of his thought. It leaves no room for any doubt about the important influence that the Muslim Philosopher had on the greatest of all Catholic theologians.

6) Muhammad ibn Musa al-Khwarizmi

Early Life:

Muhammad ibn Musa al-Khwarizmi was a Persian mathematician, astronomer, astrologer geographer and a scholar in the House of Wisdom in Baghdad. He was born in Persia of that time around 780. Al-Khwarizmi was one of the learned men who worked in the House of Wisdom. Al-Khwarizmi flourished while working as a member of the House of Wisdom in Baghdad under the leadership of Kalif al-Mamun, the son of the Khalif Harun al-Rashid, who was made famous in the Arabian Nights. The House of Wisdom was a scientific research and teaching center.

Contributions and Achievements:

Al-Khwarizmi developed the concept of the algorithm in mathematics (which is a reason for his being called the grandfather of computer science by some people).

Al-Khwarizmi’s algebra is regarded as the foundation and cornerstone of the sciences. To al-Khwarizmi we owe the world “algebra,” from the title of his greatest mathematical work, Hisab al-Jabr wa-al-Muqabala. The book, which was twice translated into Latin, by both Gerard of Cremona and Robert of Chester in the 12th century, works out several hundred simple quadratic equations by analysis as well as by geometrical example. It also has substantial sections on methods of dividing up inheritances and surveying plots of land. It is largely concerned with methods for solving practical computational problems rather than algebra as the term is now understood.

Al-Khwarizmi confined his discussion to equations of the first and second degrees. He also wrote an important work on astronomy, covering calendars, calculating true positions of the sun, moon and planets, tables of sines and tangents, spherical astronomy, astrological tables, parallax and eclipse calculations, and visibility of the moon. His astronomical work, Zij al-sindhind, is also based on the work of other scientists. As with the Algebra, its chief interest is as the earliest Arab work still in existence in Arabic.

His most recognized work as mentioned above and one that is so named after him is the mathematical concept Algorithm. The modern meaning of the word relates to a specific practice for solving a particular problem. Today, people use algorithms to do addition and long division, principles that are found in Al-Khwarizmi’s text written about 1200 years ago. Al-Khwarizmi was also responsible for introducing the Arabic numbers to the West, setting in motion a process that led to the use of the nine Arabic numerals, together with the zero sign.

Of great importance also was al-Khwarizmi’s contribution to medieval geography. He systematized and corrected Ptolemy’s research in geography, using his own original findings that are entitled as Surat al-Ard (The Shape of the Earth). The text exists in a manuscript; the maps have unfortunately not been preserved, although modern scholars have been able to reconstruct them from al-Khwarizmi’s descriptions. He supervised the work of 70 geographers to create a map of the then “known world”. When his work became known in Europe through Latin translations, his influence made a permanent mark on the development of science in the West.

Al-Khwarizmi made several important improvements to the theory and construction of sundials, which he inherited from his Indian and Hellenistic predecessors. He made tables for these instruments which considerably shortened the time needed to make specific calculations. His sundial was universal and could be observed from anywhere on the Earth. From then on, sundials were frequently placed on mosques to determine the time of prayer. The shadow square, an instrument used to determine the linear height of an object, in conjunction with the alidade for angular observations, was also invented by al-Khwarizmi in ninth-century Baghdad.

While his major contributions were the result of original research, he also did much to synthesize the existing knowledge in these fields from Greek, Indian, and other sources. A number of minor works were written by al-Khwarizmi on topics such as the astrolabe, on which he wrote on the Jewish calendar. He also wrote a political history containing horoscopes of prominent persons.

Death:

Muhammad ibn Musa al-Khwarizmi died in c. 850 being remembered as one of the most seminal scientific minds of early Islamic culture.

7) Omar Khayyam

Lived 1048 – 1131.

In the year 1072 AD, Omar Khayyam documented the most accurate year length ever calculated – a figure still accurate enough for most purposes in the modern world. Khayyam was an astronomer, astrologer, physician, philosopher, and mathematician: he made outstanding contributions in algebra. His poetry is better known in the West than any other non-Western poet.

The man himself remains something of an enigma. Different biographers have documented him as a fun-loving, wine-drinking agnostic; a closet Zoroastrian; a Sufi Muslim; an orthodox Sunni Moslem; and a follower of Ancient Greek philosophy. All agree that he was an outstanding intellectual.

Beginnings

Omar Khayyam was born on May 18, 1048 in the great trading city of Nishapur in northern Persia. Today the city is in Iran. Omar’s father was Ebrahim Khayyami, a wealthy physician. Omar’s mother’s name is not known. Some authors have written that Omar’s father earned a living making tents, because Khayyami means tent-maker. However, although many English-speakers are named Smith, it does not mean their fathers spend their days hammering hot metal on an anvil.

Omar’s family were Muslims. His father seems to have been relaxed about religion, employing a mathematician by the name of Bahmanyar bin Marzban, a devotee of the ancient Persian religion of Zoroastrianism, to tutor Omar. Bahmanyar had been a student of the great physician, scientist, and philosopher Avicenna, and he gave Omar a thorough education in science, philosophy, and mathematics. Khawjah al-Anbari taught Omar astronomy, guiding him through Ptolemy’s Almagest.

In his early teens Omar worked in his fathers’ surgery learning about medicine.

Omar Khayyam celebrated his eighteenth birthday in 1066. In that same year, Halley’s comet appeared in the heavens, William the Conqueror’s Norman Army invaded England, and Omar’s father Ebrahim died. A few months after Ebrahim’s death, Omar’s tutor Bahmanyar also died.

It was the end of an era in Omar Khayyam’s life. It was time to put his family’s affairs in order and move on.

Samarkand

Omar Khayyam joined one of the regular caravans making a three month journey from Nishapur to the great city of Samarkand, which is now in Uzbekistan. Samarkand was a center of scholarship, and Khayyam arrived there probably in 1068, aged 20.

In Samarkand he made contact with his father’s old friend Abu Tahir, who was governor and chief judge of the city. Tahir, observing Khayyam’s extraordinary talent with numbers, gave him a job in his office. Soon Khayyam was given a job in the king’s treasury.

While living in Samarkand, Khayyam made a major advance in algebra.

Omar Khayyam’s Contributions to Science

Algebra

At high school, we learn about equations of the form ax2 + bx + c = 0; these are called quadratic equations. Cubic equations are of the form ax3 + bx2 + cx + d = 0. Naturally, cubic equations are harder to solve than quadratics.

Khayyam conjectured correctly that it is not possible to solve cubic equations using the traditional Ancient Greek geometrical tools of straightedge and compass. Other methods are required.

At the age of 22, in 1070, Khayyam published one of his greatest works: Treatise on Demonstration of Problems of Algebra and Balancing. In it he showed that a cubic equation can have more than one solution. He also showed how the intersections of conic sections such as parabolas and circles can be utilized to yield geometric solutions of cubic equations. Archimedes had actually started work in this field over a thousand years earlier, when he considered the specific problem of finding the ratio of the volume of one part of a sphere to another. Khayyam considered the problem in a more general, methodical way.

Khayyam’s solutions avoided negative coefficients and negative roots because negative numbers were not acknowledged in Islamic mathematics. (Some cultures, however, had incorporated negative numbers into mathematics – for example Brahmagupta had introduced negative numbers into Indian mathematics 400 years earlier.)

Although Khayyam’s achievement was magnificent, he was personally disappointed that he needed to utilize geometry to solve cubic equations – he had hoped to discover an algorithm using only algebra.

Treatise on Demonstration of Problems of Algebra and Balancing established Khayyam as a mathematician of the first rank, and his reputation spread quickly throughout Persia.

Following Khayyam’s work there was little significant progress on cubic equations until, in 1535, Niccolo Tartaglia found general solutions for all cubic equations.

Khayyam’s algebra was not the system of letters and signs we use today. His algebra was expressed in words. So, where today we write:

Solve for x: x2 + 6 = 5x

Khayyam wrote: What is the amount of a square so that when 6 dirhams are added to it, it becomes equal to five roots of that square?

Linking Algebra and Geometry

Algebra and Geometry were successfully linked by Pierre de Fermat and René Descartes in the 1600s, resulting in the modern x-y coordinate system.

Khayyam’s work with cubics had made him certain that algebra and geometry were linked, and he cited Euclid’s Elements to support the idea:

Whoever thinks algebra is a trick in obtaining unknowns has thought it in vain. No attention should be paid to the fact that algebra and geometry are different in appearance. Algebras are geometric facts which are proved by Propositions 5 and 6 of Book 2 of Euclid’s Elements.

Length of Year

In 1073, Khayyam received an invitation to the Persian city of Isfahan, capital of the Seljuk Empire, to prepare a calendar that would work in an orderly way and be accurate forever – this was an era in which year lengths were regularly changed.

Khayyam’s invitation came from the two most powerful men in the Seljuk Empire, of which Persia was part: these were Malik Shah, Sultan of the Great Seljuq Empire, and Nizam al-Mulk, his vizier.

Khayyam recruited other talented scientists to accompany him to Isfahan in 1074. There he was paid an extraordinarily high salary and enjoyed a privileged lifestyle. Malik Shah paid Khayyam to found an observatory with an initial aim of making observations of the heavens for 30 years, during which time Saturn, the most distant planet then known, would complete an orbit.

Ptolemy’s universe – the model accepted by Omar Khayyam.

During his time in Isfahan, Khayyam measured the length of a year – to be specific the tropical year length – with remarkable accuracy and precision.

Khayyam found that 1,029,983 days made 2,820 years. This gives a tropical year length of 365.2422 days to seven significant figures. Although it has become fashionable to quote more decimal places than this, Khayyam’s input of 1,029,983 days contains seven significant figures, so it is unreasonable to quote more than this number of significant figures in the calculated year length.

Today we know that the length of a tropical year actually changes by as much as 30 minutes from year to year. The average tropical year length quoted today is 365.242189 days, which to seven significant figures is 365.2422 days – exactly the figure Khayyam arrived at almost a thousand years ago. The length of a tropical year is also increasing very slightly as time passes, although not enough between Khayyam’s era and our own to be noticeable on a scale of seven significant figures.

Malik Shah introduced Khayyam’s new calendar in the Seljuk Empire on March 15, 1079. It was used until the 20th century.

The Parallel Postulate

The 13 books of Euclid’s Elements published in about 300 BC were probably the most influential books in the entire history of mathematics. The Elements had been built on five geometric postulates – in other words five things that were assumed to be true about geometry: for example, all right angles are equal to one another.

The fifth of Euclid’s five postulates was the parallel postulate. The parallel postulate proved to be a source of puzzlement, irritation and joy for mathematicians for millennia. The joy was usually short-lived, belonging to mathematicians who thought they had proven the postulate only to be disappointed when an error was identified in their ‘proof.’

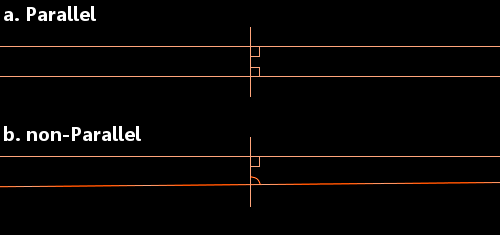
Euclid had considered a straight line crossing two other straight lines. He looked at the situation when the interior angles (shown in the image below) add to less than 180 degrees. In these circumstances, he said that the two straight lines will eventually meet on the side of the two angles that add to less than 180 degrees.

a. When each angle is 90 degrees, the lines are parallel.
b. If one or both of the angles is less than 90 degrees, the lines will meet.

Since almost the day Elements was published, mathematicians had been trying to use Euclid’s first four postulates to prove the parallel postulate. They were doomed to fail. We now know that it is impossible to prove the parallel postulate using Euclid’s other postulates.

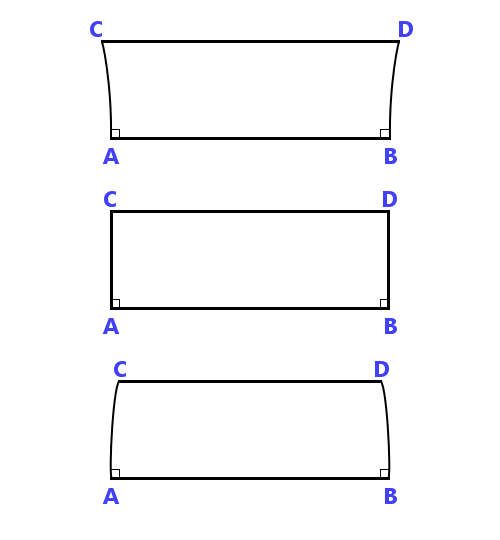
Omar Khayyam’s attempt was interesting. In his Explanations of the Difficulties in the Postulates in Euclid’s Elementshe asks his readers to consider a straight line AB:

He then asks his readers to consider two equal lines that are perpendicular to AB and sees three possible arrangements, which can produce four-sided figures:

He then refutes the possibility that angles C and/or D can be anything other than right-angles and in the image above only the central option is possible. So, he believes he has proven the parallel postulate. In fact, he has not done so, all he has done is stated it in a different way.

What is interesting to historians of mathematics is that in Khayyam’s ideas – shown roughly in the images above – they can see the first glimmers of non-Euclidean geometry.

Some Personal Details and the End

Full details of Khayyam’s personal life are not known. He is believed to have married and had at least one son and one daughter.

In 1092, Malik Shah and his vizier both died – the first probably by poisoning, the second by assassination. Khayyam went into hiding during the resulting power struggle. His survival depended on lying low. He had been Malik Shah’s personal physician and become his close personal friend – which had made him enemies – and Khayyam’s poetry suggests his behavior may not have been devoutly religious – and this had also made him enemies. Khayyam actually published no poetry in his lifetime. Some of his musings would potentially have endangered his life.

After the power struggle, it took about 20 years for Khayyam to be fully rehabilitated and for him to emerge again, at 64 years of age, in the company of powerful people. However, he refused to teach. One of his poems suggests why this might be:

The secrets which my book of love has bred,
Cannot be told for fear of loss of head;
Since none is fit to learn, or cares to know,
Tis better all my thoughts remain unsaid.

Omar Khayyam died at the age of 83 in his hometown of Nishapur on December 4, 1131. He was buried in a tomb whose location he had chosen in an orchard where blossom would fall twice a year.